The Complexity Dynamics of Magic Cubes and Twisty Puzzles

Chris King

13 Oct 2019 PDF

Fig 1: A selection of magic cubes and twisty puzzles used in the discussion of this article. (1) A Rubik 4x4’revenge’. (2) A Moyu 4x4 axis 4x4 cube shape mod. (3) A Dayan Shuang Fei Yan (cool flying swallow) cube. (4) An MF8 sun cube. (5) MF8 crazy unicorn cube. (6) MF8 windows grilles cube. (7) MF8 4-layer elite skewb. (8) MF8 ‘twins’ version of the edge-turning Skewby Copter plus. (9) Rubik 3x3 (10) Rubik 5x5 ‘professor’. (11) MoYu stickerless 9x9. (12) Jiehui ‘evil eye’ rhombic dodecahedron. (13) Alexander’s Star great dodecahedron. (14) Shengshou Megaminx dodecahedron. (15) LanLan face-turning octahedron. (16) DaYan corner turning octadedron. (17) Qiyi Qiming pyraminx face/corner turning tetrahedron. (18) Shengshou Mastermorphix (3x3 metamorphix) edge-turning tetrahedron. (19) Diang Sheng blade 3x3 cube shape mod. (20) Fang Cun Ghost Cube 3x3 shape mod. (21) 3x3 picture cube. (22) Square-1 cube. (23) Curvy Copter cube.

History:

In the mid-1970s, Ern_ Rubik designed his 3x3x3 "Magic cube" (B_vös kocka in Hungarian) as a way of solving the structural problem of rotating the parts independently without the entire mechanism falling apart. He did not realise that he had created a puzzle until the first time he scrambled his new cube and then tried to restore it. The Pyraminx was made and patented by Uwe Mèffert in 1981. The original Skewb is a shape modification of a Pyraminx invented by Tony Durham and marketed by Mèffert. Robert Webb designed the corner-turning Dino Cube in 1985. The original prototype was made entirely out of paper and first marketed with images of dinosaurs on the faces. The master and elite versions (8) now have distinct additional transformations distinct from an nxn pyraminx. The Megaminx dodecahedron, or Hungarian Supernova was invented in 1982 by Ferenc Szlivka. In the same year, a slightly different design was made by German mathematician Christoph Bandelow and produced by Mèffert. Alexander's Star was invented by US mathematician Adam Alexander in 1982. It contains only edges in 6 colour pairs for the 12 faces and is equivalent to solving only the edges of a six-color Megaminx. The Square-1 layered shape-shifting puzzle with non-cubic scrambling was invented by Karel Hr_el and Vojt_ch Kopsk_ around 1990. The edge-turning Helicopter Cube was invented by Adam G. Cowan in 2005 and the curvy, plus and skewb versions by Tom van der Zanden.

Fig 2: Four core cube designs and their rotations: The 2x2 face-turning Rubik Pocket cube, the slant-turning Skewb,
the corner-turning Dino cube and the edge-turning Helicopter cube.

Puzzles 3, 5 and 6 all contain similar corner-turning moves to the Dino cube, in addition to other move types. The Dino Cube is one of the easiest puzzles to solve since: (a) Each move only affects a small corner collection, so it is easy to solve one part of the puzzle without disturbing what is already solved. (b) Each piece only has one possible orientation, so if it is in the correct position, it correctly oriented. These features also appear in the composite cubes.

With the advent of computer programmed 3D printers, it became possible to design custom twisty puzzles enabling the development of much more complex and diverse designs. Many of these, such as the elite Skewb (7) and Skewby Copter plus (8) have subsequently moved into mainstream mass-produced items. The master Skewb, for example, was invented by Katsuhiko Okamoto in 2003, remaining a single copy until mass produced in 2011. The Elite Skewb (7) was designed by Andrew Cormier in 2009 with a 3D printed version by Mohammed Badir and mass produced by MF8 in 2018 and the Skewby Copter plus by Diogo Sousa and mass produced by MF8 in 2016.

Videos and articles exploring 3D printed design can be found here, here and here. The result is an active race, among puzzle designers to define the cutting edge of the art.

Fig 3: Computer design tutorial (Grégoire Pfennig), pre-production composite image of the MF8 Twins cube (8) Mar 2019 (now produced) and a cutaway view of the structure of the MoYu 9x9 cube (11).

Complementing the mass-produced items available, from US puzzle shops to Aliexpress, is a diverse community of puzzle building enthusiasts who frequently market their creations in boutique outlets, as illustrated below. These give expression to the potentially unbounded limits of human creative ingenuity.

Fig 4: Custom designed cubes: (a) David Pitcher’s Andromeda plus jumbling cube based on the geometry of the pentagonal icositetrahedron sharing properties with 3 and 8 in fig 1. (b) Greg’s Daffodil cube. (c) Diogo Sousa’s Bubblarian massively corner-turning dodecahedron (d) The Gigshexaminx ingeniously made by cutting down a Masterkilominx (4x4 corners-only megaminx) into cubic symmetry. (e) Grégoire’s world record holding 33x33 cube available for 3D printing pre-order for €15,200. You can see Greg assembling it by hand here. (f) David Pitcher’s Star of the Seven, RCP’s Duelling tetrahedra and David Pitcher’s Crazy Daisy.

Groups, Commutators, Orbits and Algorithms

Twisty puzzles are structures composed of geometrical corners, edges and faces which remain intact under a system of rotations in three dimensional space. The overall geometry of the structure is often a regular 3D solid such as a cube, or polyhedron, but the operations may not conform to the geometry of the whole structure. For example, the three cubes in fig 2 have three different sets of rotational operators the first 6 on cubic faces, the second and third 4 tetrahedral axes and the fourth having 8 oblique axes of edge rotation.

It is also possible for a given puzzle’s rotational core to adopt multiple structural and thus morph into several structure geometries (fig 8) and even for a puzzle to be able to be interpreted in terms of two inconsistent geometries. For example, the Mastermorphix, (fig 1 (18), is both an edge-turning tetrahedral version of the curvy copter cube (23) and is a rotational morph of the standard 3x3 face-turning Rubik cube (9).

The rotations form a group under composition (performing one after the other). A group is a set with an associative binary operator, where every pair of elements c can be multiplied to form p*q, there is an identity (staying still – nil rotation) and every element p has an inverse p’ = p^{-1 (}the reverse rotation). However, in contrast with multiplication of numbers, where 3*2 = 2*3 = 6, groups don’t have to be commutative, so in many groups p*q ≠ q*p.

Fig 5: A match box ends up with different orientations if the order of rotations is reversed.

Rotation matrices do not compose commutatively if their axes or or orientations are different. This is a basic property of matrix multiplication since:

.

In the case of the 3x3 cube, we can describe the group G in terms of six 90^o rotations of the six faces
G = {L, R, U, D, F, B). We don’t need to include the centre slice rotations because we can keep the centres fixed. The identity I represents standing still and each rotation R has an inverse R’ going in the opposite direction. G also contains a subgroup G2 consisting of each of the 180 ^o double rotations G2 = {L2, R2, U2, D2, F2, B2), where R2=R*R.

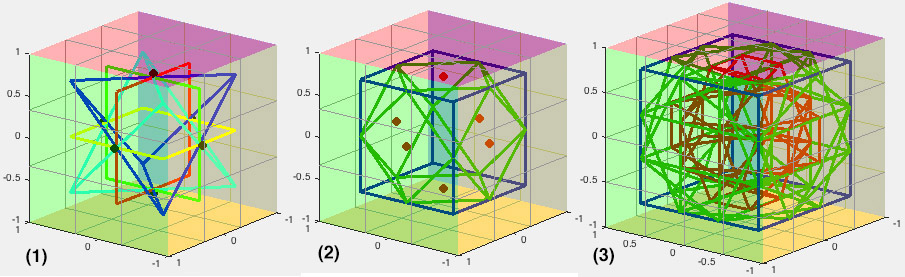
Fig 6: (1) Orbit set of the 3x3 Rubik under the 180 ^o rotation sub-group G2 contains 2 complementary braided tetrahedral corner orbit sets (cyan and blue) and 3 braided edge orbit sets (red, yellow and green). By contrast, the full 3x3 90 ^o rotation group G. (2) has fully entangled edge and corner orbit sets. The central slice moves in (1) & (2) can be factored out because the centres can be assumed fixed because they are equivalent to a pair of face moves and a reorientation of the whole cube. (3) The full group for the 4x4 cube is fundamentally more complex in its entanglements, because all edge pairs can be mixed and the inner slice moves cannot be factored out because the four centres in each face can also be arbitrarily mixed.

The orbit of a puzzle centre, edge, or corner is the set of other locations it can be carried to by the composed rotations. In some puzzle groups the orbits are braided into discrete interlacing subsets, rather than one tangled whole, so that several pieces are confined to distinct braids and remain relatively ordered, while in other groups the orbits are chaotically entangled in the sense that any type of piece can end up in any of the possible positions for that type – a state of maximal entropy or disorder. For example, the 3x3 Rubik has both its edge and corner orbit sets fully entangled in G but has three braided edge orbit sets and two braided corner orbit sets in G2.

All twisty puzzles are an exercise in unravelling disorder, so braided orbits are more amenable to intuitive solutions that exploit their symmetries, while entangled orbits require stringent algorithms to avoid re-mixing the orbits. Consequently, it only takes a few moves to get a puzzle scrambled in a way which takes a much larger number of moves to solve.

Key to finding moves which avoid re-scrambling solved parts of the puzzle are the commutators. Since the group is non-commutative, the closest elements to the identity are those that correspond to the discrepancy between p*q and q*p, for example pqp’q’ = pqp^-1q^-1 = (pq)(qp)^-1. These often move only a few pieces because, for the rest, the rotations cancel out. In all twisty puzzles the commutators are the key to solving the endgame. In the case of the 3x3 Rubik, FRF’R’ cycles 3 edges and flips and rotates two pairs of corners. More generally a derived commutator is any expression where the net power of each rotation is zero modulo 4, since 4 90^o rotations are a complete revolution and thus the same as standing still, for example the derived commutator RUR’URU2R’ of fig 7 permutes edges and corners only on the top face. One can also combine a sequence of rotations r, which move pieces into the domain of a commutator c to form a compound commutator rcr^-1.

Fig 7: (1) The elementary commutator FRF’R’ swaps two pairs of corners also rotating them and cycles thee edges. (2) The derived commutator RUR’URU2R’swaps and rotates diagonal corners and cycles three edges on the top face. Differing powers of 2 or 3 of these combined with additional moves can be used to cycle edges only, corners only or rotate corners, enabling the fin al layer to be solved without scrambling the reminder. (3) The elementary G2 groups braided orbits make it much easier to solve, even using only G2 moves, although the elementary commutator cycles two sets of 3 corners plus 3 edges, so is not amenable to separate edge and corner moves on powers, but the compound move U2,R2,F2,U2,F2,R2,U2,F2 can be used to swap pairs of edges.

For example, the layer method for solving the 3x3 Rubik is:

(a) To make free rotations to bring the bottom layer (e.g. white) edges to form a cross.

(b) Pair up the bottom layer corners with their second layer edges, making suitable rotations, using the top level as workspace.

(c) Apply the basic commutator combined with additional moves to position and rotate the corners and edges e.g. using the basis commutator powers of 2 on edges and powers of 3 on corners.

Fig 8: Example algorithms for solving the 3x3 cube.

For solving the last layer, there are a number of additional algorithms, for example

1. U R U' L' U R' U' L cycles LUB, RUB and LUF corners anticlockwise with the inverse cycling clockwise

2. B2 D' (F R F' R')³ D B2 swaps corners BUL <-> BUR & FUL <-> FUR

3. The pair of algorithms rotate corners, as long as one of each is applied, as each scrambles inversely

L D2 L' F' D2 F clockwise on UFL, e.g. followed by U F' D2 F L D2 L' U' anticlockwise on UFR

4. F2 U L R' F2 L' R U F2 cycles edges UF, UL and UR clockwise, with the inverse cycling anticlockwise.

5. F R' F' R' F2 L D R D' L' R' F2 R2 flips two edges FU & RU.

6. F2 D' F' D F' R2 B U' B' R2 swaps the ULF-URF corners, and the LU-FU edges.

Notably: 1, 2, 3, 5, have net power zero and so 1, 3, 5 are derived and 2 is a compound commutator.

In addition, for supercubes such as (21), where the centres have orientation, example additional algorithms are:

1. (R U R' U) ⁵ rotates the U centre 180^o.

2. F B' L R' U D' F' U' D L' R F' B U rotates the U centre 90 ^o clockwise and the F centre 90 ^o anticlockwise.

The total possible number of configurations is 8! x 3⁷ x 12!/2 x 2¹¹ ~ 4.32 x 10^19, since there are 8! ways to arrange the 8 corners, 3 ways to arrange 7 of the 8 corners (the last is locked to the 7), 12!/2 ways to arrange the 12 edges (half of 12! because edges must be in an even permutation when the corners are) and 11 of the 12 edges can be flipped independently. If centre orientation is also counted, as in the picture cube (21), there are 4⁶/2 ways to orient the centres since an even permutation of the corners implies an even number of quarter turns of centres as well. Since we have factored out 3 rotations of the last corner, 2 or the even edge permutations and 2 for the last edge flip there are actually 3.2.2=12 times as many possible arrangements of the disassembled pieces than the rotations provide. There are thus 11 additional sets of ‘shadow’ configurations forming unsolvable positions in the orbit sets.

God’s algorithm is the procedure to bring back Rubik’s Cube from any random position to its solved state in the minimum number of steps. Complementing this is the notion of the diameter, the minimum number of moves that can get any position to the solution. If one counts the number of distinct positions achievable from the solved state using at most 17 moves, it turns out that this number is smaller than 4.3_1019, giving 18 as a lower bound.

In 1992 Dik Winter established a 20 face turn (either 90 or 180 turn) solution for the 'superflip' (fig 9) where all edges are correctly positioned but flipped. The most scrambled state is thus far from random! In 1995 Michael Reid proved that this solution was minimal. A slightly different position was found with a minimum number of 26 quarter turns or 20 face turns. The following superflip sequence has the minimal 20 moves in the face turn metric, though it requires 28 quarter turns: U R2 F B R B2 R U2 L B2 R U' D' R2 F R' L B2 U2 F2. This one has 24 quarter turns (but 22 face turns): R' U2 B L' F U' B D F U D' L D2 F' R B' D F' U' B' U D'. When the superflip is composed with the "four-dot" or "four-spot" position, in which four faces have their centers exchanged with the centers on the opposite face, the resulting position may be unique in requiring 26 moves under the quarter turn metric.

Fig 9: The 3x3 superflip in both a cube simulation and the Mastermorphix, wher, despite there being three ‘face pieces’ to a tetrahedral face, the actual cubic face fig 14(18) has 4 ‘faces’ as actual edge pieces. Because these pieces have only one colour but a different shape when flipped, we get a single coloured ‘flower’.

Finding an upper bound requires a different kind of reasoning. Most algorithms take between 50 and 100 moves. A breakthrough, using descent through nested sub-groups was found by Morwen Thistlethwaite. Details were published in Scientific American in 1981 by Douglas Hofstadter. Thistlethwaite's method differs from layer algorithms and corners first algorithms in that it does not place pieces in their correct positions one by one. Instead it works on all the pieces at the same time, restricting them to fewer and fewer possibilities until there is only one possible position left for each piece and the cube is solved, by working successively down through subgroups of G:

By 1980 Thistlethwaite had established that his algorithm could solve any position in 52 moves (Kaur 2015). You can access a Matlab version of Thistlethwaite’s algorithm extended by the reduction method (see below) to the 4x4 case.

In 1992 Herbert Kociemba improved Thistlethwaite’s algorithm by reducing it to a two-phase algorithm requiring only the subgroups G₀, G₂, and G₄. A freeware version is available from Kociemba’s home page. Using Kociemba’s ideas, Michael Reid announced in 1995 that he had improved the upper bound to 29 face turns. There is an open source GCC version downloadable here.

At about this time, Richard Korf (1997) introduced a new approach. A GCC open source version of this algorithm can be found here. Instead of using a fixed algorithm, his strategy simultaneously searched for a solution along three different lines of attack. IDA* is a depth-first search that looks for increasingly longer solutions in a series of iterations, using a lower-bound heuristic to prune branches once a lower bound on their length exceeds the current iterations bound. It works roughly as follows. First he identified a number of sub-problems that are small enough to be solved optimally:

1. The cube restricted to only the corners, not looking at the edges

2. The cube restricted to only 6 edges, not looking at the corners nor at the other edges.

3. The cube restricted to the other 6 edges.

The number of moves required to solve any of these sub-problems is a lower bound for the number of moves you will need to solve the entire cube.

Given a random cube C, it is solved as iterative deepening. First all cubes are generated that are the result of applying 1 move to them. That is C * F, C * U, … Next, from this list, all cubes are generated that are the result of applying two moves. Then three moves and so on. If at any point a cube is found that needs too many moves based on the upper bounds to still be optimal it can be eliminated from the list.

Although this algorithm will always find optimal solutions, it is not known how many moves this algorithm might need. On average, his algorithm appeared to solve the cube in 18 moves. There was, however, no worst-case analysis, and so the upper bound held still at 29. Note also that this method uses methods requiring complex tables that would remain opaque to a human solver,

In 2006, Silviu Radu reduced the upper bound to 27. The next year, Gene Cooperman brought it down to 26 (Kunkle, & Cooperman 2007). Tomas Rokicki (2007, 2008, Rokicki et al. 2014) then entered the picture, reducing the upper bound to 25 in March 2008. Working with John Welborn, he had it down to 22 by August 2009. Finally, in July 2010, Rokicki announced an upper bound of 20, the established value of the lower bound and therefore the long-sought-after value of God’s number (van Grol 2010). Evolutionary methods have also been applied (El-Sourani, Hauke, & Borschbach 2010).

Other advanced methods for rapid speed-solving of the 3x3 such as CFOP, Petrus, ZZ and Roux abound among speed cubing communities. Often after solving the first 2 layers F2L, these separate the final layer into orienting the last layer OLL and then permuting git PLL. Another major question has been to find out whether God’s algorithm is an elegant sequence of moves that can be easily performed by humans or an arcane program requiring brute force computation.

Runs of Thistlethwaite's algorithm quickly display the relationship of the number of moves required to scramble compared with the larger number of those needed to solve. They also establish as little as 7 moves can result in a complete scramble:

Scramble (15 moves): B2,R2,F2,R2,B,L2,R',U',L2,D2,B2,R,U2,L2,D

Solution (30 moves): R,D,F2,L',U',F',R',B2,D2,L',F,L,F2,L',D2,L,D2,L,D2,L',F2,U2,F2,D2,R2,F2,U2,F2,U2,D2

Scramble (7 moves): L2,U,L2,U',F2,L2,R

Solution (31 moves): U',F2,U,L,U2,F,U2,L2,U2,L2,F,L',F2,U2,L,B2,L',F2,L2,R',U2,L2,F2,R2,B2,L2,B2,U2,B2,R2,D2

Now if we restrict to scrambling and solving using only 180^o turns (our group G2 in figs 6, 7 and Thistlethwaite’s G₃), we find that the algorithm can solve in fewer moves than the scramble indicating intrinsic ordered simplicity of the solution which needs only the G₃ component:

Scramble (15 moves): B2,U2,B2,D2,L2,B2,R2,L2,U2,R2,D2,B2,U2,L2,R2

Solution (11 moves): L2,B2,L2,U2,F2,U2,L2,D2,F2,R2,F2

Scramble (15 moves): F2,R2,U2,D2,F2,R2,L2,F2,L2,F2,U2,B2,R2,F2,R2

Solution (7 moves): L2,B2,R2,U2,D2,F2,D2

Scramble (30 moves): U2,D2,F2,D2,R2,F2,B2,R2,D2,F2,D2,L2,U2,F2,L2,D2,U2,D2,R2,F2,D2,U2,L2,R2,B2, U2,F2,B2,F2,U2.

Solution (8 moves): L2,D2,B2,L2,D2,L2,U2,F2

One can contrast this with the much longer, manual solution by Antonio Vivaldi using the algorithm (4) in fig 7. Because of the braided corner orbit sets, the corners can quickly be placed by basic rotations, but the edges then need to be positioned by pairs of parity flips using algorithm (4).

Overview of the Puzzle Set

To explore the complexity dynamics, let’s first examine the representative puzzles in Fig 1, and provide some pointers to the solutions. This will both serve to enable anyone to actually solve the puzzles themselves and provide a view on the varieties of dynamics they induce.

A: The Rubik NxNxN Series

The straight Rubik face turning cubes fig 2 (1) and fig 1 (9, 1, 10, 11) show the 2x2, 3x3, 4x4, 5x5 and 9x9, form a series leading up to the very large 33x33 example fig 4(d). The 2x2 is effectively identical to the 3x3 with edges and centres removed and uses a reduced set of the 3x3 algorithms. All the NxN for N even have no fixed centre determining the relative orientations of the coloured faces, so the correct spatial parity has to be determined by inspecting the corners before beginning.

Number of Configurations

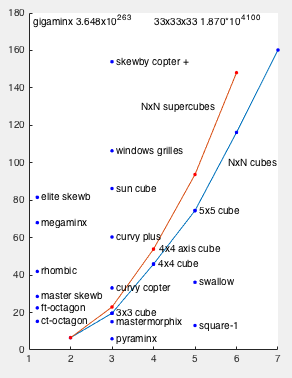Fig 10: Log₁₀ plot showing diverse geometries and super-exponential rise of configurations for NxN cubes (blue) and super-cubes (red).

As a result of their varying parities, the number of configurations for odd and even NxN cubes differs. Let n=(N-1)/2 for N odd, n=N/2 for n even:

Odd N: 12 edges with 2 orientations each (12! * 2^11), 8 corners with 3 orientations each (8!/2 * 3^7), n-1 orbits of 24 edge wings ((24!)^(n-1)), and n^2-n different orbits of 24 centers, all of which are made up of 4 pieces each of 6 types ((24!/4!^6)^(n^2-n)).

Thus the total number of positions is 12! * 8! * 24!^(n^2-1) * 4!^(-6n^2+6n) * 2^10 * 3^7. For this family the existing puzzles are n=1 (3x3x3, with 4.325 * 10^19 positions), n=2 (5x5x5, with 2.829 * 10^74 positions), and n=3 (7x7x7, with 1.950 * 10^160 positions up to n=16 (33x33x33 fig 4, with 1.870*10^4100 positions).

Even N: 7 corners with 3 orientations each (7! * 3^6), n-1 orbits of 24 edge wings ((24!)^(n-1)), and n^2-2n+1 orbits of 24 centers, each of 4 pieces in 6 colors ((24!/4!^6)^(n^2-2n+1)).

The total number of positions here is 7! * 24!^(n^2-n) * 4!^(-6n^2+12n-6) * 3^6. The puzzles in this family that have been constructed are n=1 (2x2x2, with 3.674 * 10^6 positions), n=2 (4x4x4, with 7.401 * 10^45 positions), and n=3 (6x6x6, with 1.572 * 10^116 positions).

For the supercube cases, where all pieces are distinct and orientable, we have 12! * 8! * 2^(-n^2+n+21) * 3^7 * 24!^(n^2-1) for N odd and 7! * 24!^(n^2-n) * 3^6 * 2^(-n^2+2n-1) for N even.

There are two approaches to solving the NxNxN series. The first is a layer by layer approach as for the 3x3, which is okay up to the 5x5 but becomes increasingly more tedious. The 4x4 introduces parity issues both due to 3D spatial parity inversion and due to odd permutations generated by the slice moves so that a single pair of edges can become flipped or two edges become swapped. The second type of parity issue also occurs in the 5x5 in which just one edge can be flipped.

The favoured approach, which becomes ever more essential as n increases, is reduction of the larger cube to a scrambled 3x3 by (1) Positioning the centres, (2) pairing up the edges and then (3) solving the resulting layout as a 3x3. This requires only learning a few simple positioning algorithms. Since the centres of a given face are identically labelled the solution needs only to position each type of centre into any of its positions, so for very large n cubes, the centres can be placed in sets of convenient patterns. Pairing the edges into a solution set is more demanding although there are fewer of these as they are distinguishable.

4x4 Reduction: For the 4x4 reduction we have the following algorithms. Here small letters are the slice moves and capitals the face moves.

Fig 11: (1-5) Stages in reducing a 4x4 to 3x3 illustrated from the solved cube. (6) Reduced cube.

The reduction begins with moves which position centres in the correct face, allowing the other pieces to remain scrambled and then pair the adjacent edges together without positioning them on the cube, leaving a 3x3 solution to complete:

1. To fix centres on the U face, apply U to put an incorrect piece in Ulb from one of the side faces,

r U r' moves Frd -> Ufl also placing the incorrect Ulb in Fru. Non-centre pieces are freely scrambled.

Alternatively, r2Ur2’ moves Drb -> also placing the incorrect Ulb in Drf.

2. If you find your parity is wrong at this point, u2 R2 L2 u2 will swap the L and R centre sets keeping other centres fixed.

3. To pair edges, find any pair of edge pieces that have the same colours, but are not yet adjacent. Use face moves only to place one at FDr and the other at FUl. Find the piece with colours matching FUr, and place it at FRd using only face moves. If this is not possible, then put any unmatched piece there. Then r U' R U r' U' R' U will pair UFl and DFr to UFl and UFr. Notice this disturbs a third edge set and the piece moved is flipped so try to position it flipped correctly to pair with the UFl piece. If there are no unmatched pieces, you have to swap FUr and FDr but not move anything else (4). I if needed, U2 r U2 r U2 r U2 r U2 r U2 will swap FUr <-> FDr and FUr <-> FDr to free 3 edge pairs. Both these moves cancel out all slice moves, so the centres remain in position.

4. To resolve edge parity issues: r' U2 l F2 l' F2 r2 U2 r U2 r' U2 F2 r2 F2 double flips UFl & UFr edges

5. F l B L2 B' l' B L2 B' F' l' D2 U2 r' D2 U2 l' d2 l2 d2 also swaps edges UFl and UBl

The extension of the Thistlethwaite algorithm to reducing the 4x4 results in much longer sequences of moves to find the solution:

Cube Dimension: 4 Solving Method: 423T45

Scramble (7 moves): l2,U,l2,L',b2,l',d

Solution (160 moves): U2,B,l,U,l',U2,r2,U,r2,d,R,d',R,B,u,R,u',L',R,d2,R,d2,D,l,D,l',D',L2,b,D,b',D2,b,D,b',D,F,u,L,u',L',F2,u,L,u',L,B2,d,L,d',L2,F,u2,B,u2,B',F2,u2,B,u2,D2,L,B2,U2,u,F',U,F,u',F',U',D,L',R2,D',L,B2,b,L',B,L,b',L',B',L,R,F',U2,L,F2,r,R2,D',R,D,r',D',R',U',D,L',B2,U,F',L',F,U2,u,F',U,F,u',F',U',F,R2,F,D,L,D,l,U',L,U,l',U',L',U,L2,R',U,L2,U2,L,D2,F,L2,R,F',R',B2,R,D2,R',B2,R,U2,L,R2,U2,B2,U2,B2,L2,U2,R2,B2,R2,U2,B2.

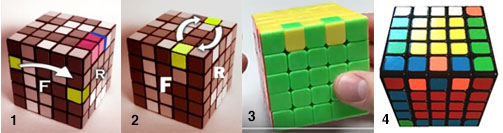
Fig 12: (1,2) Edge-pairing moves on the 5x5 cube, (3) double edge flip, (4) parity flip on nearly completed reduction.

5x5 Reduction: The situation for the 5x5 reduction is similar but more complex to complete. Here are sample algorithms that need to be combined with care to move affected pieces clear of rescrambling. Capitals followed by w moves both slice and face together. The centres are now of two types: edge and corner:

1. To fix the edge centres use u2 F u2 to send Bu -> Fl and u F u' to send Ru- > Fl.

2. To fix the centre corners use u' R' u R' u' R2 u to send Ruf -> Fur

and u2 B' u2 B' u2 B2 u2 to send Bur -> Fur.

3. To pair edges (1), find any central edge piece and any lateral edge piece that has the same colours, but which are not yet adjacent. Use face moves only to place them in the m and u layers respectively. Make sure that the central edge piece is oriented in such a way that a move of the u layer would line the two pieces up correctly. Use any face moves to place an unmatched edge piece at the FLu position. Give priority to those triplets where the FLu piece has the same colours as its adjacent central edge now at FRm, but where the central edge is upside down. Use u' R U R' u keeping URm unpaired, or d R' D' R d' keeping DRm unpaired. If you cannot find any other unmatched edge, then do the sequence R2 u R2 u R2 u R2 u R2 u R2 and try again. Rotate u, and m to line the three edges up at the FR position.

4. To swap opposing edges (2) UB & DB use l' U2 l' U2 F2 l' F2 r U2 r' U2 l2. They should be unpaired.

5. To flip two edges (3) use r' U2 l F2 l' F2 r2 U2 r U2 r' U2 F2 r2 F2.

6. To swap FUl & BUr as well as FUL & BUR use F2 Rw U2 Rw U2 Rw' F2 Rw' U2 Rw' U2 Rw U2 Rw' U2 Rw2.

7. To correct edge parity (4) use Rw U2 Rw' U2 Rw U2 Rw U2 Lw' U2 Rw U2 Rw' U2 x' U2 Rw2.

A solution video can be found here. You can see a variety of pattern algorithms here.

NxN Reduction

Generalizing to larger Rubik cubes involves using the principles of the above reduction methods, as summarised below. The best way of exploring this process in detail is by watching tutorial videos of the 5x5x5 and 9x9x9 solutions both by cubing bear to show how the 5x5 solution extends to the 9x9.

Fig 13: Steps in a 9x9 solution. (Left) U (top) is being used to fill F (bottom) with centre pieces in vertical strips, and contiguous patterns. Edge pairing (top right) involves temporary displacements of the centres and algorithms (bottom right) to complete the last stages.

(a) Centres: For very large cubes, one can use two adjacent faces, say U and F building horizontal rows of a given colour in U and then rotating them and slotting rows vertically into the F face, also rotating and moving patterns that will add centre pieces without disruption.

1. Solve the centres of two opposite faces say F and then D, using U as a workspace, by matching up all of the centre pieces of that colour.

2. Solve another two adjacent centres on the puzzle using the freedom of the 4 unsolved centres on the puzzle.

3. Use commutators and puzzle knowledge to solve one of the last two centres, leaving the last centre solved.

(b) Edge sets The edge sets although fewer are all distinguishable and their rearrangements have to preserve the centres over the process, so their matching up is more demanding:

1. First 8 sets Temporarily disturb the solved centres to match edge pieces and complete 8 edges, placing each solved edge on to the top and bottom layers of the cube.

2. Last 4 edge sets – After realigning the centres, use algorithmic combinations to complete the final 4 edges

(d) 3x3 Solution as above.

B Rubik Shape Mods

Puzzles 2, 18, 19 and 20 in figs 1 and 8 illustrate a variety of so-called ‘shape mods’ of the face-turning 3x3 and 4x4 cubes using the same internal mechanisms and having essentially the same solutions, with the exception that the centre pieces are generally distinguishable and have defined orientations, so additional algorithms are required to place these pieces correctly. Some other pieces may lose their orientations reducing the total number of conformations.

Fig 14: (18, 19 & 20) ‘Shape mods of the 3x3 cube. (2) is a 4x4 shape mod. Each are oriented to show their planes of rotation are identical to those of the 3x3 and 4x4 cubes. Their solutions are thus also identical, except that the centre pieces now have an identified orientation, (and position in the 4x4 case), as does the picture cube fig 1 (21). However, when scrambled, these puzzles present a serious pattern recognition problem, as their scrambled shapes (right) are almost unrecognisable. Notice that the ‘faces’ are now corner sets (2, 19 & 20), or edge sets in the case of (18). (2) and (19) are regular oblique axis morphs, while (20) is irregular and its pieces are entirely asymmetrical. (18) Can be represented either as a face-turning 3x3 cube mod or a tetrahedral edge-turning relative of the curvy copter (23) with similar jumbling moves. Notice all the rotating sets preserve the arrangement of centres edges and corners on the corresponding 3x3 or 4x4 face. On (19) for example, the centre is a corner and the actual corners are the large corner piece and 3 small triangles, while the actual edges are the 2 large faces and the 2 bi-coloured edges, preserving the 3x3 Rubik face arrangement.

These puzzles can present a major pattern recognition task for the solver because the pieces can only be identified by their shape. The image of (2) above shows that a face turn consists an oblique corner slice and although the ‘face’ has complete homology with the 4x4 cube in terms of having four centres, four edge pairs and four corners, many of the boundary pieces are of only o e colour with no indication of where they match up colour-wise as is the case with two-coloured edge pieces on the cube. Each ‘face’ actually consists of a triple of colours each of which is shared with other ‘faces’. The slice moves are also very problematic to keep track of as there are no obvious landmarks and the shape and colours keep transforming.

(2) and (19) form regular symmetric morphs of the 4x4 and 3x3 cubes with (19) stretched into a prismatic parallelohedron form. These referred to generally as ‘axis’ cubes because the axes of symmetry of the cube has been reoriented off the axes of the rotation operations. Their ‘faces’ are thus regular, and the pieces on each of the 6 ‘faces’ are alike. By contrast, the Ghost cube (20) is asymmetrically reoriented, so that all the pieces are different, as well as being in only one colour, so recognition depends entirely on shape. There are a variety of other 3x3 shape mods, including the Fisher and Mirror cubes and other geometries.

The solution methods for the 3x3 cases are the same as the 3x3 supercube above. For layer solutions of the 4x4 axis cube see: 1. Antonio Vivaldi’s tutorial Part1, Part2 , 2. L M Cubing’s Tutorial , 3. Seppomania’s Parity fix.

The Mastermorphix (18) is exceptional because it is both a tetrahedral shape morph of the 3x3 cube and is also a tetradedral realization of the edge-turning ‘copter’ cubes under 180^o rotations, complete with corresponding jumbling moves arising from the 90^o rotations. Also, because some of the pieces are indistinguishable or don’t have visible orientations in both (18) and (19) above, the the total number of combinations is less than that of the 3x3 supercube, as we shall show below for Mastermorphix. Notice that all the actual cube edge pieces, as illustrated in fig 14(18), are the tetrahedral faces, the ‘edges’ are actually the centres and the corners of a face actually consist of two corners and two ‘centers’. Hence the super-fip algorithm fig 9 flips all the faces on a given side to make a ‘flower’.

C: The Diverse Geometries of Face and Corner-turning Twisty Puzzles

We will consider only the analogues of the 3x3 cube in detail, to elucidate how varying geometries introduce new dynamical systems into the mix.

Fig 15: (1) Pyraminx can be thought of as either corner-turning or face-turning but only corner turning commutators are useful for solving the puzzle. (2) The elementary adjacent corner commutator cycles only 3 edges. The algorithm R U R' U R U R' U cycles three edges on the same level.

Pyraminx Tetrahedral Series

Pyraminx can be thought of as either a corner-turning or a face-turning puzzle, or both, but the face-turning moves involve scrambling a majority of the pieces, so the only elementary commutators of interest arise from compositions of second-layer corner moves, the first layer being trivial rotations of a single corner piece. In addition, the three faces next to the corner are not separated by any moves so are manufactured as a single block.

All turns create even permutations, so every orbit has permutation parity. Investigation of the elementary commutator fig 15 (2) shows that the only permutations generated are a 3-cycle of the edges, meaning this is purely an edge-moving puzzle solved by the elementary commutator.

Excluding the 3^4 trivial tip positions, there are 4 corners each with 3 orientations (1 * 3^4), 6 middle edges with 2 orientations each (6!/2 * 2^5), n-1 orbits of 12 edge wings ((12!/2)^(n-1)), a total of floor((n-1)^2/3) orbits of 12 centres, in 3 centres for each of 4 colours ((12!/3!^4)^(floor((n-1)^2/3))), and ((n-1)^2 mod 3) orbits of 4 centres ((4!/2)^((n-1)^2 mod 3)).

Thus the number of positions is 6! * 2^(5-n-((n-1)^2 mod 3)) * 3^4 * 12!^(n-1+floor((n-1)^2/3)) * 3!^(-4floor((n-1)^2/3)) * 4!^((n-1)^2 mod 3). For the 3x3 pyraminx with n = 1, there are 7.558 * 10^7 positions, counting both tips and corners, 933120 if only corners are counted and 11520 if neither these trivial rotations are counted. With the 4x4 master version with n = 2, we get 2.681 * 10^15 positions, still not approaching the complexity of the 3x3 cube.

The solution requires only positioning and orienting the tips and corners correctly in terms of the corner colours using elementary rotations and then applying either of the 3 cycle algorithms in fig 15.

Mastermorphix

The edge-turning Mastermorphix provides an alternative to the corner vs face turns of the pyraminx which incorporates further interesting properties of the tetrahedral geometry, which we will investigate in the section on edge turning puzzles. In particular the 90^o rotations become the equivalent of jumbling moves.

The overall polyhedral symmetry is of an edge-turning tetrahedron, while the underlying rotations when the slices to permit rotations are included are identical to the face-turning 3x3 cube. In the tetrahedral geometry these correspond to edge-turning jumbling moves, so we have two dual interpretations of the orbital dynamics.

Fig 16: The same algorithm L2 R2 F2 B2 U2 D2 results is a dual pair of symmetries in the Mastermorphix and 3x3 cube.

The number of moves is as follows: There are four corners and four face centers. These may be interchanged with each other in 8! different ways. Although the puzzle is a 3x3 cube shape mod, there are only 3^4 ways for the corners to be oriented, since the face centre orientations (which also correspond to the cube corners) are not visible. There are 12 non-central face pieces. These can be flipped in 2^11 ways and there are 12!/2 ways to arrange them. The three non-central face pieces of a given colour which correspond to cube edges are indistinguishable. Since there are 6 ways to arrange the 3 pieces of the same colour and there are 4 colorus, there would be 2^11_12!/(3!)^4 possibilities for these pieces.

Hence the total is 8! * 3^4 * 12!/2 * 2^11 /( 3!)^4 = 1.236 * 10^15.

The solution the same as the 3x3 supercube except the ‘center’ corners have no preferred orientation..

Megaminx Dodecahedral Series

The Megaminx is an edge-turning puzzle with very similar dynamics to the 3x3 cube. All turns create even 5-cycles, so every orbit has permutation parity. The elementary commutator on adjacent faces, like the cube, swaps two pairs of corners and cycles three edges, as shown in fig 16 (1). The solution proceeds as with the 3x3 cube first placing the correct edges in the bottom layer, then positioning corner pieces with their adjacent edge pieces in the five adjacent and then five upper layers using the same techniques as the cube.

Fig 17: (1) The elementary commutator on adjacent faces swaps 2 pairs of corners and cycles 3 edges. (2) Swapping an edge pair and a corner pair. (3) Cycling three edges (also moving corners). (4) Cycling 3 corners. (5) Rotating 2 corners.

Algorithms as shown in fig 16 can then be used to complete the solution in the top layer. The algorithms are:

(1) F R F’ R’, (2) F U R U' R' F' (3) R U R' U R U3 R' U (4) L' U2 R U'2 L U2 R' U'2 and (5) (R' D' R D)^2 U (D' R' D R)^2 U'.

The Kilominx, which lacks the edges is solved by considering only the corners.

There are 30 edges with 2 orientations each (30!/2 * 2^29), 20 corners with 3 orientations each (20!/2 * 3^19), n-1 orbits of 60 edge wings ((60!/2)^(n-1)), and n^2-n orbits of 60 centers, in 12 colors of 5 pieces each ((60!/5!^12)^(n^2-n)).

Hence the total number of positions is 30! * 20! * 60!^(n^2-1) * 5!^(-12n^2+12n) * 2^(28-n) * 3^19. This gives the Megaminx, with n = 1, 1.007 * 10^68 positions and the 4x4 Gigaminx with n =2 having a whopping 3.648 * 10^263 positions. The Kilominx with corners only can be calculated by eliminating the edge permutations, and Alexander’s star by eliminating the corner permutations.

Jaap’s solution page.

Alexander’s Star

The Alexander Star is equivalent to the Megaminx without the corner pieces, so is solved the same way considering only the edges and edge algorithms.

We choose one pair of pieces and fix one. There are two identical pieces, so we can fix each position exactly two ways, so we have to divide the result by 2. The number of positions is approximately (29*(28!/2!^14) * 2^28)/2 or 7.243 * 10^34.

Face-Turning Octahedron

The Face-turning octahedron brings with it some surprising new features and displays features of triangulely tiled polyhedral like the icosahedral puzzles. The rotational axes are at the centre of the triangular faces and do not pass through any piece. Consequently, the puzzle has to be constructed as a puzzle within a puzzle, with an internal spherical twisty mechanism fig 17 (right) holding the edges in fixed orientation in relation to the mechanism, with the centres and corners floating latched under these. This means (a) that all the pieces are permuted, but also (b) that the edges orientation is determined by their position as in a corner-turning Dino cube. You can see this if you try to flip a piece by consecutive rotations, where successive faces are connected in fours at each corner, not the threes in the cube and Megaminx, so an edge can’t be flipped using adjacent faces .

Fig 18: Left four: On the face-turning octahedron, there are cycles of three different lengths in the elementary commutator R U R’ U’ here U=red, R= green). Two views are shown so all the permuting pieces can be seen. Two pairs of corners are swapped and rotated 90^o (cyan) three edges are cycled (yellow) and two sets of five faces are cycled. Top left-centre the commutator squared rotates corners by 180^o. Bottom left-centre: the algorithm R U R’ U R U R’ cycles 3 edges on a face (R=green U=red). Centre-right: The octagon is a puzzle within a puzzle. The edges are mounted on a smaller twisty puzzle, so they can orbit since none of the pieces in the main puzzle are on the centre of rotation. Right top: R' U L' U' L U' R U swaps two corners (U=yellow R=green L=blue). Right bottom: (r U r' U' r)^5 swaps two centres (R=green U=red).

If you tile the faces of the pieces in a dark-light checkerboard, none of the moves mix the colours. Therefore the corners have only two orientations, and the edges only one, behaving like a dino cube corner rotation. Furthermore, the centres split in two sets that don't intermingle. Consequently we have 6 corners with 2 orientations, 12 edge pieces, and two sets of 12 centre pieces. This gives a combined upper bound of 6!*2^6*12!*12!^2 arrangements. However only an even number of vertex pieces are flipped (2), the vertex permutation is even (2), the edge permutation is even (2), the centres come in identical triplets (3!8) and the orientation of the puzzle does not matter (12 rather than 24 since we fix the orientation of the puzzle by fixing one unique corner or edge, which has 12 possibilities).

The total number of positions is therefore 6!*2^3*11!*12!^2 / 3!^8 = 3.141*10^22.

If you examine the elementary commutator R U R’ U’ as shown in fig 18 (left) you find that, as usual two pairs of corners are swapped and three edges cycled, however there are two sets of 5 cycles among the centres of two colours, in fig 18 red/grey and green/purple. This means that unlike the cube and megaminx, where comm^6 = I here it takes comm^60 = I, because it takes com^4 to make 2 180^o rotations of the corners and 4, 3, and 5 are relatively prime.

So the most straightforward method of solution is as follows.

(1) Solve the edges, keeping the colour scheme consistent with the corners, as the ‘dino’ moves enable one to do this without re-scrambling, as the final orbits resolve.

(2) Solve the corners using the commutator as in fig 17.
You can flip a pair of adjacent corners using R' U L' U' L U' R U, or alternatively do R U' R' U R' L R L' (fig 18).

(3) Position the centres using a commutator 5-cycle as shown in fig 18. Using (r U r' U' r)^5 (where r means a turn of the middle R layer clockwise, as viewed from the R face) will move only 2 centre pieces of different colours (fig 17).

Antonio Vivaldi has a tutorial on the solution. Jaap’s solution page.

Fig 19: (1) The elementary commutator cycles three edges. (2) R U R' U R U2 R' cycles 3 edges. (3) R' U R U' R' U2 R U R' U' R U2 swaps 2 edge pairs. (4) R' U F' U' F U' R U or R D' F D2 F' D R' D2 flips 2 corners.

Corner-turning Octahedron

This works just like the Pyraminx, but now there are parities involved, since a turn creates edge 4-cycles.

To solve:

1. Position and rotate the tips and the corners so the colours match the corners.

2. Position the edges using R U R' U R U2 R' to cycle three edges RU -> BU -> LU -> RU

and/or R' U R U' R' U2 R U R' U' R U2, to swap two pairs of edge pieces UF <-> UL & UR <-> UB.

3. To flip RU & FU edges and restore parity, use R' U F' U' F U' R U or R D' F D2 F' D R' D2.

There are 12 edges with 2 orientations each (12!/2 * 2^11) and 6 fixed corners with 4 orientations each (1 * 4^6), The total number is thus (12!/2 * 2^11) *(1 * 4^6) = 2.009 * 10^15.

Evil Eye Rhombic Dodecahedron

The “evil eye” so called because some versions have coloured centre piece recessed at the vertices introduces yet further variations to the theme. Again the axes of rotation are the same as the cube and the rhombic dodecahedron is a form of cube in which the faces are elevated into pyramids.

There are five layers, 2 face layers two lateral slices and one centre slice as in a 5x5 cube, however, here the face moves all commute with one another because their pieces do not intersect, but the lateral slice moves scramble 8 faces. The eyeless evil eye shown consists only of ‘edge’ and ‘corner’ pieces. The edges occur in pairs of a given colour. We name the rotations as in a 5x5 cube.

Fig 20: (1) Two views of the solved ‘evil eye’ puzzle looking down on the U and F faces for comparison with the following moves. (2) r U r' U r U2 r' permutes corners in a 5 cycle. (3) F' r U' r' F moves an edge between adjacent faces. (4) r U2 r' U r U' r' U2 r U' r' moves an edge between faces (5) r U r' U' r' F r2 U' r' U' r U r' F' swaps two edges and two corners. (6) r U r' U r U2 r' U2 r U r' U r U2 r' U2

The sketch solution is as follows:

1. Position the ‘eyes’ correctly in relation to the corner colours if the puzzle has them. This can be done by making rotations involving the slice moves and at the end, using f2 u' l r' f2 l' r u' f2 to 3-cycle the last 3.

2. The puzzle can then be solved up to the centre slice by elementary moves. Algorithms are then needed as in fig 20 (2) to (4) to move pieces into position without single slice moves re-scrambling the puzzle.

3. The final face can be aligned using a combination of the moves in fig 20 (5) and (6).

4. If a parity error arises due to two identical edge pieces remaining swapped, a single edge from an identical pair can be moved using (3) or (4) and the remaining scrambled elements resolved.

There are 24 corners and 24 edges, both of whose permutations are even, and the edges are in indistinguishable pairs so the total number of positions is 23!*24!/2^2/2^12 = 9.789* 10^41.

A tutorial solution is available here.

D The Skewb Series

Fig 21: (1) Scrambled Elite (2) The three skewb cuts showing the 2 slice moves and two large face moves. (3) Elementary commutators permuting the Elite edges and faces in a way which can reduce it to a Master Skewb. (4) the green face and white centre (upper image) are folded to the left using a lower move and then a commutator to swap diametrical pairs in a cube face.

Reducing and Solving the Elite Skewb

The Skewb series presents a cube whose rotational axes are on the corners but whose cuts are deep enough to affect all 6 faces symmetrically. The original Skewb (fig 2) had a single central cut slicing the cube faces into a diamond with four triangular corners. The master version has two cuts and is analogous to the 3x3 cube and the Elite version with three cuts is both analogous to the 4x4 cube with two large face moves and two narrow slice moves. The Elite thus has the moves of both the original Skewb and the Master Skewb, so can perform all three solutions.

However, from here things differ from the 4x4 cube, because the edge wings and face pieces of the Elite Skewb can only be placed by moves involving global commutators and the face pieces come in 3 incompatible types, so commutators have to be found to cycle each of the piece types in a non-scrambling sequence. The solution is made all the more challenging because every move involves all 6 cube faces, requiring a lot of 3D observation.

We use the notation ufr(1, 2) = R(1, 2), ufl(1, 2) = L(1, 2), dfl(1, 2) = D(1, 2), ubl(1, 2) = U(1, 2) where R is the Elite/Master face turn, R1 is the first slice, and R2 the second (equivalent to the inverse of the first slice of the opposing corner rotation).

Reducing the Elite to the Master Skewb

The commutators below provide a sequence of moves which can rearrange the smaller pieces into groups forming the edge and faces of the Master Skewb shown in fig21(4, 5):

1. R1 L1’ R1’ L1 cycles small square faces in 2 3-cycle sets as shown in fig21(3a).

2. R1 L2’ R1’ L2 cycles small edge wings in 2 3-cycle sets as shown in fig21(3b). The mirror formulae e.g. R2 L1 swap the complementary edge wings.

3. L1 U’ L1’ U cycles 3 inner faces and 5 outer faces as well as pieces permuted by 1, 2 above.

4. L2 U’ L2’ U cycles 3 outer faces and 5 inner faces as well as pieces permuted by 1, 2 above.

The strategy thus consists of (a) using 3, 4 and their variants to group inner and outer faces together and then using 1, 2 and their variants to correctly place the small faces and edge wings together to form the Master edge complexes.

Antonio Vivaldi’s tutorial on the Elite Skewb, and solving the Master Skewb.

Solving the Master Skewb

The technique depends of first arranging the corners with the correct orientation and one set of edge complexes in place on the first layer to set the overall parity of the corners correctly and then to use entirely commutators of one sort or another to arrange the remaining edges centres and faces. These may change the rotations of the upper corners but they will retain parity and can be corrected at the end.

1. Use lower corner rotations to place the four edge complexes onto the first face in the correct colour order in relation to their corners.

2. Use upper rotations to place and orient the upper corners correctly. Correctly rotate each by moving up and rotating. Then move this piece back down and restore a neighbouring lower corner by an inverse move if it was displaced by the rotation.

From now on all moves have to be some sort of commutator aba'b' to preserve the parity of the corners, but top layer corners are now allowed to move because commutators preserve corner parity.

3. Place second level edge complexes, by moving each complex out by a before doing b' then a' carries the edge complex back into position and b moves it up: a b' a' b.

4. Align top layer edge complexes by permuting UL>UR>UF>UL by R' L R L'.

5. Cycle centres F>U>L>F by R U' R' U This will also move faces and corners.

6. Swap FUL and FDR faces using a = R U' R' U D' U D U'. To swap face pieces between adjacent or opposing faces, apply r a r' where r is a rotation from a neighbouring side (or two rotations from both neighbouring sides to swap between opposing faces), as in fig21(4).

7. Swap and rotate opposing pairs of corners (UFL <> UBR. and DBL <> DFR)

using b = (R U' R' U)^3 (or equivalently (U' R U R')^3 ).

You can use r b r' where r rotates the closer (UFL) corner to the same top colour as the further corner (UBR).

8. Rotate corners DBL antic and DFR clock by (U' R U R')^3, (U R' U' R)3.

The Bearded Cubing tutorial used for my solution. Jaap’s solution page.

For the standard Skewb, there are 4 fixed corners with 3 orientations each, 4 free corners with 3 orientations each and 6 face pieces giving a maximum of 6!*4!*38 positions. This limit is not reached because, the total twist of the corners is fixed (3), the faces must have an even permutation (2), the free corners must have an even permutation, and hence form a tetrad (2), finally because the tetrads are distinct the orientations one tetrad and the position of one of the others corners will determine the positions of the other three (3). This leaves 6!*4!*3^6/4 = 3,149,280 positions.

For the Master version again because the corner rotations move corners across face diagonals, the corner orbits consist of two corner tetrads which never intermingle. Similarly, the off-centre face pieces fall into two orbits - corresponding to the two corner tetrads. There are 8 corners with 3 orientations each, 6 face centre pieces, two sets of 12 other face pieces, and 12 edge pieces which (like the dino cube) cannot be flipped. This gives a maximum of 4!*3^8*6!*12!^3 positions.

Again this limit is not reached because: The total twist of the corners is fixed (3). The faces centres must have an even permutation (2). The edges must have an even permutation (2). The free corners must have an even permutation, and hence form a tetrad (2). The orientations of the fixed corners and the position of one of the free corners will determine the positions of the other three (3). 12 face pieces in each orbit come in six identical pairs (2!^12)

This gives a total of 4!*3^8*6!*12!^3/(2^15*3^2) = 4.225*10^28 positions.

For the Elite version there are now 2 sets of 12 edge wings with no flips in 2 orbits, 2 sets of 12 small faces in indistinguishable pairs, 2 sets of 12 outer faces, and 2 sets of 12 inner faces again in pairs:

This gives a total of 4!*3^8*6!*12!^10/(2^41*3^2) = 3.643*10^81 positions.

Fig 22: (1) Both the Mastermorphix and all forms of helicopter puzzle enable jumbling moves, the former by making a 90^o half-turn and the latter by a turn of about 71^o on two faces, as shown. (2) Without jumbling, both puzzles have braided edge orbits that become entangled only when the jumbling moves are taken into account, where two pairs of pieces are exchanged between distinct orbits. The corner orbits are two tetrads in the former and a cuboidal entangled orbit in the latter. (3) Both the Mastermorphix and the plus series of copter cubes such as the Skewby-copter plus and twins (illustrated) can exchange corner pieces and centre faces. (4) The MF8 Helicopter dodecahedron.

D: Edge turning puzzles

There are a series of puzzles in which rotations occur on axis symmetries protruding from the edges of a give geometrical polyhedron. These include the Cubic Curvy Copter, Skewby Copter Twins fig 2 (23,8), the tetrahedral Mastermorphix (18), and other variants such as the MF8 Helicopter dodecahedron fig 22. These share a two-tiered structure of moves, where the primary (non-jumbling) moves are 180^o rotations of an edge. While the edge pieces now have fixed positions, this results in a set of face orbits which are braided into a number of separate strands, as shown 2 in fig 22 for the Curvy Copter and Mastermorphix. Complementing these, there are jumbling moves shown 1 in fig 22, in which pairs of edge-turning assemblies can in turn have two face pairs swapped thus making a double exchange between the braided orbits. In the case of the cubes the corner orbits are still fully entangled (a corner can end up in any corner position) but in the Mastermorphix the non-jumbling corner moves form two tetrads, as they are transformationally equivalent to the 3x3 cube under 180^o rotations.

The Mastermorphix was first made by several producers in 2009, four years after the helicopter cube. Intriguingly the solution of the Mastermorphix as a jumbling edge-turning puzzle equates to reducing the full 3x3 cube group to the subgroup G₃ of 180^o rotations, precisely the last subgroup in Thistlethwaite’s algorithm. Thus not only is the Mastermorphix a dual between the cubic rotational geometry of the axis cuts and the overall tetrahedral geometry of the puzzle, but the edge-turning, jumbling solution is identical to the group reduction method for the cube.

Due to the braided face orbits the non-jumbling solutions are relatively easy to perform without the need for brute force algorithms, while the jumbling states can also be unwound by exchanging pairs of faces which are out of orbit.

A sketch non-jumbling solution for the Curvy Copter is as follows, where F R B L, are 180o rotations on the top layer:

1. Orient all the edge pieces on the first (initially top) face to their correct colours.

2. Flip one edge down and use the distinct orbits and non-jumbling edge rotations to move the top (say red) edge pieces onto the two face positions on the side flipped face and flip up to the top. Repeat so the top face is complete.

3. Corner-edge trios

(a) Find correct corner to match top layer, flip it to the bottom and if necessary swing the corner around the bottom (4 steps) to orient it (by four 1/3 rotations) so that a single side edge flip will place it in the top layer in the correct orientation.

(b) Move correctly coloured faces in the orbit of each adjacent face to adjacent and flip up into position, taking the corner out of the way for the second adjacent move and flip and flip the trio to the top. This completes the top half of the side faces.

(c) Now flip the cube over and use the unsolved top layer to hold pairs of side faces and flip to the appropriate side layer keeping the edge orientations correct. This will automatically leave the top faces correctly as they are the left-over pieces in the raided orbits.

4. Finalize Corners

(a) Permute top face corners in a 3-cycle holding ULF fixed using R L B L B R B L B L.

(b) Orient 3 corners holding ULF fixed using (F R B L)^6 anticlockwise or (L B R F)^6 clockwise.

Additional jumbled solution moves:

(a)If a triangle is out of orbit, swap with an adjacent top face colour by flipping both to opposite sides and jumbling.

(b)To flip an edge and swap two adjacent corners fig 23(a), use JR JL F, where JR is jumbling with 2 clockwise twists JL anticlockwise twists.

Fig 23: (a) Flip an edge and adjacent corners, (b,c) swapping out of orbit faces.

(c) Using R L JR F R L, swap two pairs Y, G (starred and locked in the same orbit fig 23b) and the red triangles (starred and in neighbouring orbits, but of the same colour), these initially swapped in the R L to be opposite.

(d) Use the same move (fig23c) to escape an orbit trap (the yellow and red ones, thumb and index finger in the same orbit and the two red starred ones). Use F R F R to 3-cycle faces (yellow, red as shown and green on top).

You can follow these solutions in RedKB’s non-jumbling and jumbling tutorials.

The Curvy Copter can also be put in mildly jumbled non-cubic states, which need to be resolved before the cubic solution.

However, the Skewby Copter Plus and Twins present a far more formidable challenge. Firstly, there are mixing as well as jumbling moves, in which multiple partial edge rotations can both exchange centres and corners (3 in fig 22) and virtually all successive jumbling moves can be performed with non-cubic piece colliding. The skewb moves also separate the arts of the edge complexes causing these to become scrambled. In addition Skewb moves further scramble the non-cubic conformation, requiring re-association of inverted and rotated face sections.

The solution involves first reducing the jumbled puzzle to the cube. Much of the early phase is intuitive, but involves using skewb moves with commutator-type cancellations, as well as unjumbling moves. Some of the above types of moves also apply, for example re-pairing corners with their ‘ears’ using an inverse of the move in fig 22(3) and taking a rotated centre mixed by the move in fig 22(3) in four step of edge moves around a side to re-orient it before unmixing it again. The second phase is edge reduction to reunite the pieces of the edge complexes which have been mixed by the skewb moves. Then the face pieces need to be reduced before we have a final curvy copter solution.

Fig 24: Skewby Copter Plus scrambled and partially-solved in a cubic state. The twins version

(right) has an additional cut splitting the edge assembly and enabling further skewb slices.

Bottom row: Stages in Antonio Vivaldi’s reduction to cubic shape.

The only way to come anywhere close to understanding a full solution is to follow Antonio Vivaldi, who has a suite of tutorials both, scrambling and solving the skewby copter plus in three stages: making the cube, edge reduction, face reduction and endgame.

To estimate the number of positions on the copter series of cubes, we first consider the helicopter cube, without jumbling moves (only 180^o rotations). Any permutation of the corners is possible, including odd permutations. Seven of the corners can be independently rotated, and the orientation of the eighth depends on the other seven, giving 8!_3^7 combinations. There are 24 face centres, which can be arranged in 24! different ways. But the face centres actually occur in 4 distinct orbits, each containing all colours. So the number of permutations is reduced to 6!^4 arrangements. The permutation of the face centres is even, the number of permutations is divided by 2. Assuming the cube does not have a fixed orientation in space, and that the permutations resulting from rotating the cube without twisting it are considered identical, the number of permutations is reduced by a factor of 24, because all 24 possible positions and orientations of the first corner are equivalent because of the lack of fixed centres. This gives a total number of permutations of 7!*3^6*6!^4/2 = 4.936*10^17.

When a Helicopter Cube is scrambled with jumbling moves but still retains its cube shape, then face centers do not occur in 4 distinct orbits. Assuming that the four centres of each colour are indistinguishable, the number of permutations is reduced to 24!/(4!^6) arrangements, because there are 4! ways to arrange the four pieces of each of 6 colours. This gives a total number of permutations of 7!*3^6*24!/4!^6= 1.192*10^22.

For the curvy copter with 12 edges with fixed positions these figures become:

Non-jumbling: 7!*3^6*6!^4*2^12*24/2 = 4.853*10^22. Jumbling: 7!*3^6*24!/4!^6*2^12*24 = 1.172*10^27.

Fig 25: Non-cubic positions on the helicopter cube. Order: the size of the symmetry group. Index of the symmetry group as a subgroup of the full cubic group (48 divided by the order). This is also the number of ways any particular shape with that symmetry can be oriented in space (including reflections). Shapes: the number of shapes found for each symmetry group not counting mirror images. Mirror image counts the mirror image shapes. Total is Index * Mirror image.

To count non-cube positions, we need to count all the possible shapes (ignoring the colours). Counting those shapes is tricky, since sometimes moves are blocked purely due to the shape of the pieces rather than the underlying mechanism. An analysis by Matt Galla found 14,098 shapes, or 28,055 if mirror images are counted too. Some of these have symmetry however, and therefore occur in fewer than 24 (or 48) possible orientations. Below is a breakdown. Multiplying this with the previous result gives 1.529 * 10^33 for the curvy copter.

For the curvy copter plus, allowing exchanges between corner and centre faces for the plus version would give a further 32!/4!^6 variations, giving a total of 2.105*10^60.

For the Skewby Copter Plus, we have 5 faces types each indistinguishable in 4’s of the same colour, the edges are now with two wings so 2^12 becomes 24! We still have non cubic positions and corners exchanged with edges as above The 24 centres have 3 orientations. The value would be something like 7!*3^6*(24!/4!^6)^5*24!*24*1.305*10^6*3^24*32!/4!^6 = 1.002*10^154.

E: Interacting Face and Corner Rotations: Windows Grilles and Crazy Unicorn

These two puzzles provide further examples where there are interacting orbits of differing types, in these cases involving both 3x3 cube face moves and dino corner moves and in the case of the crazy unicorn additional centre moves which link the corner and edge moves in constrained orbits which preserve the relationship between pairs of centre pieces. In both these puzzles the reduced scrambling results in solutions where the corner moves tends to resolve themselves as braided orbits come together as the last few pieces become resolved. Like the NxN cubes, we proceed by reduction to a 3x3 solution.

Fig 26: MF8 Windows Grilles (1) Corner moves, (2) Scrambled, (3) Centres reduced and edge wings aligned. (4) Edge centre 3-cycle: fru' flu fru flu'. (5) Corner edge 3-cycle R' FRU' R BRU (6) Rotation of a single corner (R BRU R' FRU)^2.

On the windows grilles, let’s define the small clockwise corner rotation by FRU and the large by fru. We proceed by first completing the centre squares, then aligning the edge complexes, first by pairing the edge wings to either side of the centre, then the lateral wings rotated by the smaller corner moves and finally the centre pieces, before moving on to the next edge assembly. These can be done using moves which don’t re-scramble edge assemblies by taking critical pieces out of the way of a given corner rotation and then at the end using a commutator on the centre pieces.

A sketch reduction is as follows:

1. Complete the centre squares by face moves and then larger corner moves to fold the correct centre triangles into place. If you get two triangles to swap on the last 2 faces, use a neighbouring complete face to bring in one triangle and swap it in the second move to reverse the 3 cycle colours.

2. We now align the edge assemblies, consisting of 2 edge corners, 2 wings and a center without disturbing the centre triangles. With a give left edge wing in UF, use FRU' U L' FRU ( L U') to move the right wing from adjacent to the FUR corner. Like the 4x4 and 5x5 cubes, face moves will not upset edge assembly alignments. Bring them together, without disruption, by first rotating the second edge wing in using fru’ moving the edge assembly out of the way by face moves and then undoing the fru to relocate the centre triangles in the above commutator.

3. To align the corner edges e.g. the right, move it to adjacent to the FUR corner, along with 2 other unaligned edge corners and twist using face moves to position it with the correct flip to rotate in correctly.

4. To align a center from UR to UF, apply FRU' FLU FRU FLU'. Make sure it is flipped correctly to rotate into position.

This will completely align the edge assemblies one by one, leaving 3 at the end to align together.

5. To align the last three edge wing pairs, arrange remaining edge sets UL, UF and UR, check a clockwise rotation of flu will align the UF pair and apply R' flu U' R U flu' R'.

6. Align the last corner edges by moving 3 bad ones to the flu corner in sequence and swapping the three corner edges until all are aligned, by applying R' fru' R bru.

7. Cycle the three centres UR -> UF -> UL via FRU' FLU FRU FLU'. If two centres are flipped you will need to do a face move to position them so that a corner rotation can flip them.

8. To rotate the single fru corner clockwise, apply (R BRU R' FRU)^2. For anticlockwise, reverse the corner moves. In some solution approaches, it is also possible to get 2-edges centres swapped although it doesn’t appear here.

RubikArt has a very good tutorial on this solved intuitively. It is in Spanish, but you can arrange YouTube to provide real-time subtitles in English and several other languages, by selecting the settings cog wheel, choosing subtitles and re-entering to select automatic translation. See in particular: 3.33 centres, 7.32 edges, 13.24 last 3 edges, 18.19 last corner edges, 19.26 3x3 solve, 22.21 last corner.

The number of moves, calculated by multiplying the 3x3 configuration by the the edge piece configurations and additional corner orientations is: 4.325*10^19*24!^4/4!^6*3/2^4 = 6.288*10^105.

Fig 27: (1) The state after an anticlockwise front corner rotation followed by a 90^o U, showing the face move has not shifted the centre pieces in the U layer. (2) A scrambled cube. (3) Centre resolved into squares with the correct triangle pieces. (4) Centre pieces fully reduced. (5) Edges aligned ready for a 3x3 solve.

Crazy Unicorn

The crazy unicorn introduces three different kinds of rotations, 3x3 face moves, corner rotations on only a single tetrad of corners (hence the unicorn) and crazy moves in the centre, where only face rotations of adjacent faces move the centre pieces, but rotation of their own face leaves them fixed, as shown for the blue piece in fig27(1).

This confines the centre piece orbits. Only the triangles and larger wedge centres are moved by corner rotations. The triangles are moved only by corner rotations, and thus have confined separate orbits. The smaller wedge pieces are confined to rotate within their adjacent face as a fixed set of four. The larger wedges are subject to both rotations and face moves of their adjacent face rotations and these preserve pairs of wedges, which move together in sequences of these two moves following identical orbits. In addition, corner moves can take place only if there are a net set of 180^o rotations of the faces, otherwise they are prevented from turning by the inverted edge sections (1, 2). These constraints make the puzzle in Antonio Vivaldi’s words “a puzzle within a puzzle”.

We use a strategy of reduction in which the centre triangles are first trivially aligned with their centres (1), to complete the squares fig27(3). We then have to rotate the faces until all the corner moves are accessible by 3x3 face moves including edge flips. We can then use commutator-like moves (3) to orbit the larger centre wedge pairs into their correct centre. The last sets of pairs will all rationalize by applying one of the moves in (3). We then pair the edges (4), using commutator-like moves to avoid scrambling existing edge pairs. One then does a modified 3x3 solve (5, 6, 7) to ensure the centres end up in the correct positions:

(1) Orient centre triangles using corner moves

(2) Set non corner centres in position

(3) Use triangle moves such as

(a) F2 flu+ frd+ F2 flu- frd- to move pairs of rotating centres into position

(b) flu- frb- U2 flu+ frb+ U2 to exchange 4 on the same level

avoiding disturbing existing pieces

(4) Pair the edges using e.g. for large piece G in fu using F2 flu- T F2 flu+ T'

where T' moves the small complement g of G into fd, avoiding breaking good pairs.

(5) Make a white cross taking an edge aside e.g. to ld, before flipping the white centres down using F2 to

join the edge to the centres with D and take them up with F2.

(6) Place the corners and middle edges as on a 3x3.

(7) Use F R U R' U' F' to permute the edges to match one centre and then repeatedly to permute the

remaining edges holding uf fixed until all match. You may need to flip some edges here.

(8) Perform the usual corner cycles and rotations to complete (R U R' U' F', R U R' U R U2 R', U R U' L' U R' U' L,

(R' D' R D)^4).

Pete’s tutorial used for my solution is here. Antonio’s tutorial is here.

E: Puzzles with Irregular Conformations: Square-1, Sun and Flying Swallow.

Square-1

Fig 28: Square-1 solved, scrambled in cubic shape and two non-cubic arrangements.

Square-1 is a cubic puzzle with top and bottom faces consisting of four corners and four edges with the edges having half the angle of the corners, separated by a middle layer with a single cut that enables ‘half’ the pieces at a time constitution a 180^o slice to be switched between top and bottom. Because the corners have twice the angle, these moves can result in a number of different geometries, with differing numbers of available slice twists, from 4 top and bottom in the cubic state down to one or two in other states. The non-cubic states comprise a variety of forms, including states with broken symmetry which can be accessed through different slices through the centre move of the odd permutation in fig (29) Some of these can be exited at only a few positions.

If we view the cube from the right hand face and define:

– = flip right half through centre section

t/b = rotate top/bottom clockwise one feasible cut line

op(k) = op^k op = move sequence

Repeated operations have some very long orbits.

(a) {(t–)(82) }visits many non-cubic states before returning to the cube. The full periodicity back to the completed cube is 4*82 = 328 since the permutation of the corners (1 - 8) and the edges (a-h) is (1728)(ag)(cd).

(b) {(tb–)(8)} returns to the cube permuted by (148)(263)(57)(afbecgdh) requiring period 3*8*8 = 192.

Fig 29: Non-cubic cascade of positions and a sample of cubic solution algorithms I originally made to solve Square-1.

The solution consists of first reducing the puzzle to cubic form using the upper sequence of moves in fig 29. Elementary rotatios followed by algorithms such as op1-10 shown in fig 29 then complete the solution.

To swap top and bottom op0={t-t(4)b(4)-t(-1)}.

To calculate the number of moves for the Square-1, there are three categories of puzzle shapes. Both layers have 4 edges and 4 corners each. One layer has 3 corners, 6 edges, the other 5 corners 2 edges. One layer has 2 corners, 8 edges, the other 6 corners and no edges. There are 1, 3, 10, 10 and 5 layer shapes with 6, 5, 4, 3 and 2 corners. This means there are 5*1+10*3+10*10+3*10+1*5 = 170 shapes for the top and bottom layers. The middle layer has two shapes (half of it is assumed to be fixed). This means that there seem to be 170*2*8!*8! = 552,738,816,000 positions if we disregard rotations of the layers. Some layer shapes however have symmetry, and these have been counted too many times this way.

To take account of the symmetries we can simply count the number of layer shapes differently. Instead of the numbers 1, 3, 10, 10, 5 we use the numbers 2, 36, 105, 112, 54, which are the number of shapes if we consider rotations different (e.g. a square counts as 3 because it has three possible orientations). By the same method as before we then get 19305*2*8!*8! or 62,768,369,664,000 positions. To exclude layer rotations, divide by 12^2 to get a total of 15!/3 = 435,891,456,000 distinct positions.

If instead we wish to count only all those positions where there are no corner pieces in the way of twisting the halves, then we can use the same method but counting only all the different ways each shape can be split into two halves, e.g. a square counts as 2 this time. The numbers to plug in are now 1, 12, 46, 62, and 37 which gives a total of 3678*2*8!*8! = 1.196*10^13 twistable positions.

Sun Cube

The MF8 Sun Cube and its closely equivalent cousin, the DaYan Bagua cube, extend the rotations of the 3x3 cube by providing cuts and additional pieces which also permit face rotations in steps of 45^o. This splits the edge and centre pieces, resulting in 4 pairs of left- and right-handed kite face pieces and 8 small wedge face pieces on each face of the cube. The 45^o rotations when combined on successive faces in sequence can also rapidly lead to non-cubic geometries which become bandaged, either externally, by splits which prevent rotation, or left and right handed kites which internally prevent a further rotation because their internal structures are mirror images of one another, although several 45^o rotations can cause them to become exchanged.

Fig 30: (1) A sun pattern on the sun cube generated by repeated applications of F+ B- R2 L2 U2 D2 F- B+ R2 L2 U2 D2 F2 B2. (2) Scrambled by a sequence of 45^o moves. (7) Fully scrambled stickerless version. (3) All corners on the front face by 45o twists and 180^o flips. (4) The commutator F+ R F- R’ cycles 3 small wedges and 5 kites. (6) (F+ R F- R’)^9 returns to the cubic shape cycling 5 kites. (6) The algorithm U+ Li U- 2F Li U L 2F U+ L U- cycles three small wedges and also makes 3x3 moves. (8) The algorithm 2R U+2R D- 2R U+ 2R U- 2R U- D+ Ri cycles and unbandages three wedges, leaving a swapped corner and edge (9).

Define M+ as a clockwise 45^o rotation of face M and M- the inverse with M and M’ being the usual 90^o rotations.

We sketch a solution based on Dan Avi's tutorial below, in the following stages:

(1) Reduce to the cubic shape. Try to get a single square face. To flip an edge put it in a corner position and apply Ri Di R D repeatedly until flipped. Do the same thing to swap and edge and a corner.

(2) Unbandage the edge kites (12.38). Needs edge pieces on unbandaged DR DB and R, B faces of UR UB to apply the algorithm 2R U+2R D- 2R U+ 2R U- 2R U- D+ Ri which 3-cycles kites around the UB edge, as shown (). This leaves the cube with a corner and edge on the top and bottom swapped, so repeat Ri Di R D to regain the cube shape.

(3) Reduce the centres (21.17).

Three cycle small triangles completing the centre square, using U+ Li U- 2F Li U L 2F U+ L U-.

(4) Reduce the edges (45.21). This can be done intuitively by looking for matchable edge slices and using top and bottom 45^o turns and a 180o flip to align, using commutator like moves to keep aligned small centre wedges unscrambled to bring together misaligned kites. Join the wedges take the aligned edge out of the way and then reverse the alignment move to restore the centre wedges before restoring the edge assembly.

(5) 3x3 solve.

(6) Fix parity if necessary, resolve and complete. To swap 2 corners UFL and UFR apply U+ 2R U- Di 2F U+. Then swap the protruding corner and edge pieces top and bottom using Ri Di R D repeatedly. This may result in having to do (2) (3) and (4) over again to fix some scrambled pieces, before a final 3x3 solve.

Dan Avi’s scrambling the sun cube and tutorial and parity fix.

An approximate estimate of the number of cubic configurations of the sun cube, counting the kite face pieces of a given colour as indistinguishable is: 4.325*10^19 *(48!/(8!^6))^2 / 2 = 1.805*10^86.

Cool Flying Swallow

The Dayan Shuang Fei Yan, or Cool Flying Swallow cube has no face turns but instead four sets of three oblique face turns set in tripets around one of the tetrahedral corner tetrads, and a set of four dino corner rotations, with a slightly different curvature, intersecting the oblique rotations on the complementary tetrad. This presents a radically different situation to that found in the Sun or Skewby Copter where the non-cubic conformations tend to be obstructed and increasingly confined in their rotations and the final algorithms are performed in the cubic state. Instead it is the non-cubic states which provide the riches repertoire of algorithms.

Fig 31: (1) An oblique face rotation left and a corner rotation right. (2) Commuting oblique rotations on the same corner translocating pieces. (3) Oblique rotations and (4) Oblique and corner rotations translocating pieces across the cube. (5) Stages in QBando’s solution in order of piece type. (6) Bon Bon’s algorithms have non-cubic intermediates.

With the exception of the forbidden move combinations trying to mix moves with conflicting curvature, all the other move combinations result in transformations which can rapidly carry pieces in orbits all over the cube surface leading to a plethora of highly-scrambled non-cubic states.

QBAndo has a tutorial solving the cube, which is in Spanish, but can be viewed using English subtitles by adjusting the settings as in the Windows Grilles tutorial. A shorter explanation of the movements in English can be found here.

A suite of nine algorithm examples are also provided by Bon Bon: 0 1 2 3 4 5 6 7 8.

Because of the highly irregular conformations, it is difficult to give a precise account of the method of solution. QBAndo’s method is a form of reduction positioning the pieces in order of their type rather than face by face.

To estimate the number of cubic positions, we have 4 fixed corners and 4 floating corners with 3 rotations, 12 fixed oblique centres, 12 edges which can’t be flipped in their cubic positions, 12 triangular centres in indistinguishable pairs, 6 rectangular centres, 12 left-handed and 12 right-handed triangular faces and 12 each of LH and RH quadrilateral faces each in indistinguishable pairs, giving as a first rough approximation 4!*3^4*12!*12!/2^12*6!*(12!/2^12)^2 = 1.072*10^30 positions assuming the oblique rotations allow odd permutations. However, this is a significant order of magnitude underestimate of the total positions, because the interesting transformations arise from non-cubic positions and haven’t been counted. A rough order of magnitude estimate based on jumbling positions above would be 1.399*10^36.

References

El-Sourani N Hauke S, Borschbach M (2010) An Evolutionary Approach for Solving the Rubik’s Cube Incorporating Exact Methods C.

In Di Chio et al. (Eds.): EvoApplications 2010, Part I, LNCS 6024 80–89.

Kaur H (2015) Algorithms for solving the Rubik's cube A study of how to solve the Rubik’s cube using two popular approaches: the Thistlewaite’s algorithm and the IDA* algorithm. http://www.diva-portal.org/smash/get/diva2:816583/FULLTEXT01.pdf

Korf R (1997) Finding optimal solutions to Rubik's cube using pattern databases. AAAI/IAAI Jul 27 700-705.

Kunkle, D., & Cooperman, G. (2007, July). Twenty-six moves suffice for Rubik's cube. In Proceedings of the 2007 international symposium on Symbolic and algebraic computation (pp. 235-242). ACM.

Nelson R (2018) Abstracting the Rubik’s Cube, Math Horizons, 25/4 18-22.

Rokicki, T. (2008). Twenty-five moves suffice for Rubik's cube. arXiv preprint arXiv:0803.3435.

Rokicki, T. (2010). Twenty-Two Moves Suffice for Rubik’s Cube®. The Mathematical Intelligencer, 32(1), 33-40.

Rokicki, T., Kociemba, H., Davidson, M., & Dethridge, J. (2014). The diameter of the rubik's cube group is twenty. SIAM Review, 56(4), 645-670.doi:10.1137/140973499.

van Grol, R 2010 The Quest for God's Number Math Horizons 18/2 doi:10.4169/194762110X535961.